Delving into the realm of mathematics, let’s embark on a captivating journey to explore the complexities of the x2 y2 z2 formula. Whether you’re a budding mathematician eager to enhance your understanding or a professional seeking to delve deeper into its applications, this comprehensive article will illuminate the essence of this intriguing formula.
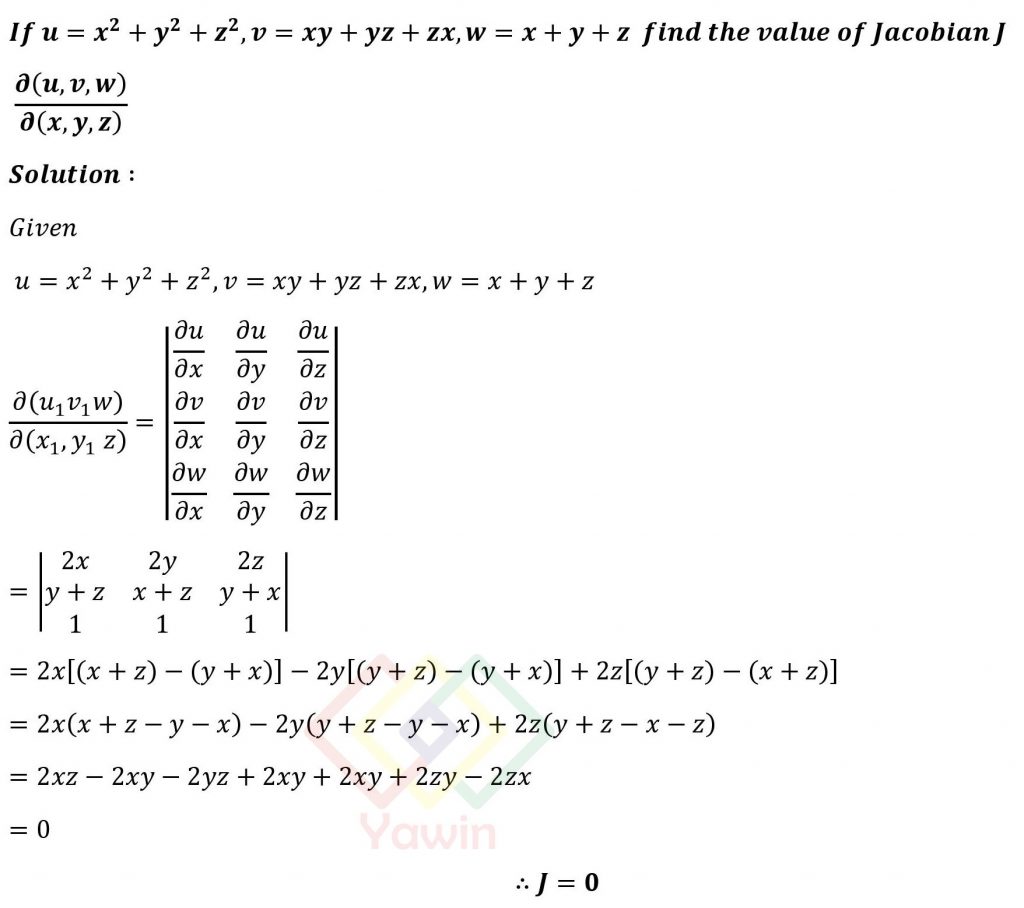
Image: www.yawin.in
Prepare to venture into the world of polynomials and equations as we unveil the secrets behind the x2 y2 z2 formula. Along the way, we will explore its history, unravel its intricate workings, and uncover its significance in various fields of science and engineering.
Delving into Polynomials and Equations
Polynomials at a Glance
Polynomials, the cornerstone of the x2 y2 z2 formula, are expressions comprised of constant coefficients and variables. These variables are raised to non-negative integer exponents. In other words, polynomials represent the sum of terms formed by multiplying variables by constants.
Consider the example of a quadratic polynomial: 4x2 – 2xy + 3y2. This polynomial boasts constant coefficients (4, 2, and 3) and variables (x and y), elevated to powers of 2 and 1, respectively.
Types of Polynomial Equations
Polynomials form the building blocks of polynomial equations, expressions that equate a polynomial to zero. Solving these equations involves determining the values of the variables for which the polynomial evaluates to zero. A prominent class of polynomial equations is quadratic equations.
Quadratic equations, typified by the form ax2 + bx + c = 0, can be solved using various techniques. The solutions to a quadratic equation determine whether the corresponding polynomial has roots (values of x where the polynomial equals zero) and how many.
Image: www.chegg.com
Unraveling the X2 Y2 Z2 Formula
Origin of the Formula
The x2 y2 z2 formula, also known as the “Perfect Square Trinomial Factorization Formula,” stems from the realm of algebra. It provides a simple yet powerful method for factorizing quadratic trinomials of the form a2 + 2ab + b2.
The Formula in Full Bloom
The x2 y2 z2 formula states that:
a2 + 2ab + b2 = (a + b)2
In this equation, the letters a and b signify variables.
Applying the Formula to Real-World Problems
The x2 y2 z2 formula finds widespread application in various scientific and engineering disciplines. Engineers leverage it for intricate calculations involving areas, volumes, and other geometric aspects. It also plays a vital role in physics and astronomy for modeling periodic phenomena like planetary orbits.
Insights from Experts and Trendsetters
Comprehensive Guide to the Formula
Tip: Seek Guidance for Deeper Understanding
Don’t hesitate to delve into textbooks, consult online resources, and engage with subject matter experts for a thorough grasp of the x2 y2 z2 formula.
Expert Advice: Apply to Various Scenarios
Practice makes perfect! Master the art of applying the formula to an array of problems, encompassing diverse areas such as geometry and algebra.
Frequently Asked Questions
Q: Does the x2 y2 z2 formula work for expressions with higher exponents?
A: No, the formula specifically applies to quadratic trinomials of the form a2 + 2ab + b2. For higher exponents, alternative approaches are necessary.
Q: Are there any limitations to the formula?
A: The x2 y2 z2 formula is only applicable to expressions that adhere to its specific structure. Outside this context, it cannot be applied.
X 2 Y 2 Z 2 Formula
https://youtube.com/watch?v=6y5b7YOUJrM
Conclusion
Through this immersive article, we have unraveled the enigmatic x2 y2 z2 formula. From its humble beginnings in algebra to its profound impact on scientific disciplines, we have illuminated its significance and provided expert insights for further exploration.
If this journey has piqued your curiosity, be sure to delve deeper into the realm of polynomials and equations. Embrace the opportunity to unravel more captivating formulas and expand your mathematical horizons. The world of mathematics awaits your eager exploration!

