A Journey to Find the Similarities

It’s the best of times when I’m curled up with a captivating novel, and the worst of times when the doorbell rings and I get lured out of the literary oasis I’d created for myself. This time, a puzzled neighbor stood at my doorstep with an arithmetic riddle, “What is the greatest common factor, or GCF, of 24 and 20?” As I delved into the world of common multiples and prime factorization, I couldn’t help but think how numbers have a strange yet enchanting way of transforming everyday interactions.

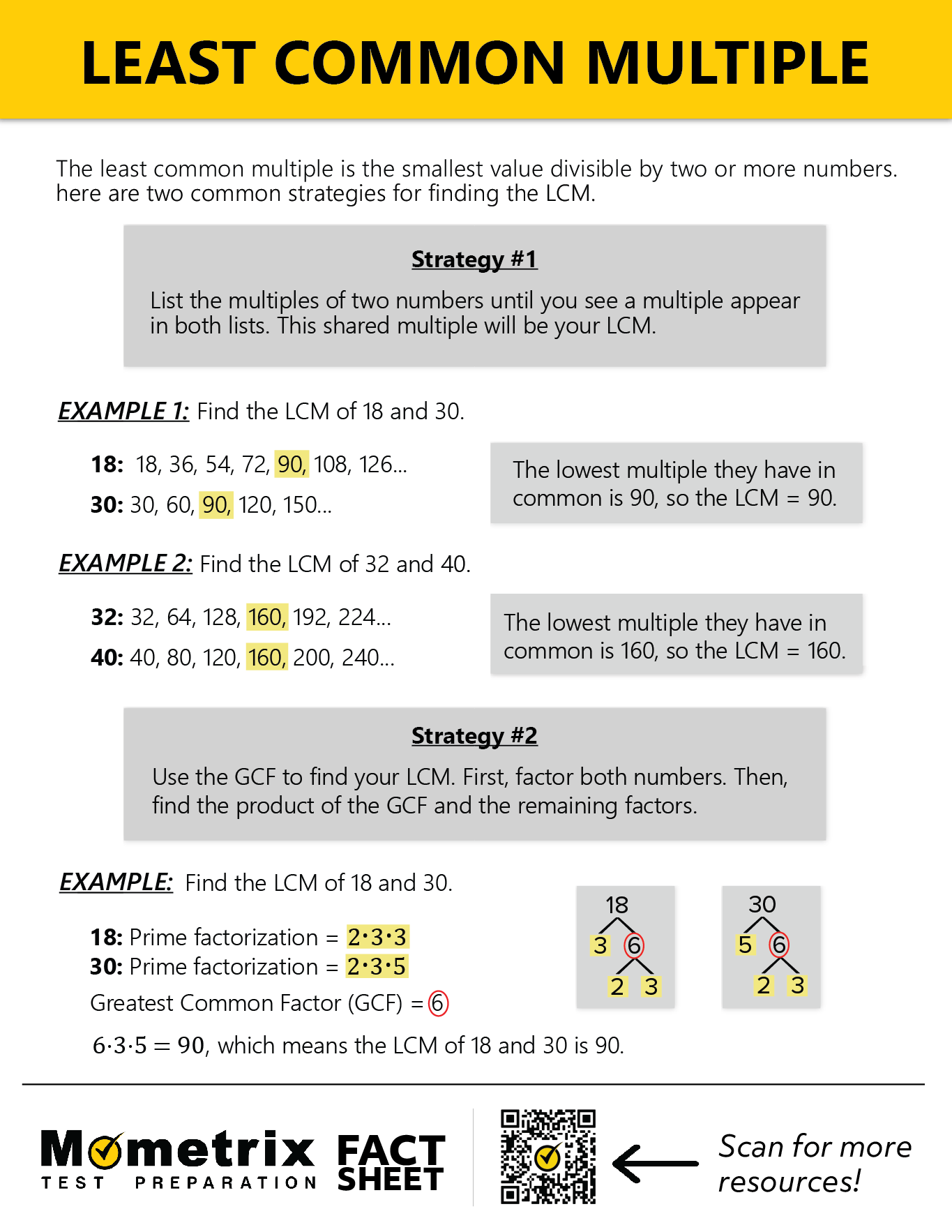
Image: www.mometrix.com

Factors and Multiples: Understanding the Basics

The greatest common factor (GCF), also known as the highest common factor (HCF), is the largest positive integer that evenly divides both given numbers. Prime factorization, on the other hand, breaks numbers into the unique combination of prime numbers that create them, similar to genetic DNA blueprints for numbers. By understanding the prime factorization of numbers, we can find their GCF and least common multiple (LCM).

Calculating the GCF Using Prime Factorization

To calculate the GCF using prime factorization, first find the prime factors of each number. For 24, the prime factors are 2 x 2 x 2 x 3, and for 20, the prime factors are 2 x 2 x 5. The GCF is found by identifying the common prime factors with the lowest possible exponents and multiplying them together. In this case, both numbers have 2 as a common prime factor. Since the lowest exponent of 2 is 2, our GCF is 2 x 2 = 4.

Exploring Greatest Common Factor Applications

The concept of GCF doesn’t just live in the realm of academic exercises. From simplifying fractions and understanding number relationships to solving real-world problems like finding the largest square tiles that can fit evenly on both a rectangular floor and a square wall, it plays a meaningful role. By mastering the GCF, we unlock a numerical key that opens doors to a myriad of possibilities.

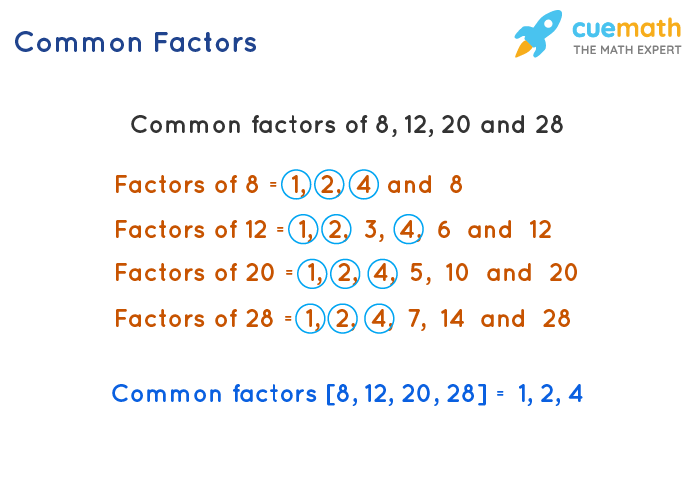
Image: www.cuemath.com

Tips and Expert Advice for Mastering GCF

• Visualize the Factors: Drawing factor trees or Venn diagrams can provide a clearer picture of the relationship between factors and multiples.
• Practice Regularly: The key to proficiency lies in consistent practice. Engage in regular problem-solving exercises to strengthen your understanding.
• Seek Expert Guidance: When confronted with a complex GCF problem, don’t hesitate to seek professional guidance from a math tutor or online forums.

FAQs on Greatest Common Factor

Q: How do I find the GCF of numbers that don’t share any common factors?
A: The GCF of such numbers is 1.

Q: Why is finding the GCF important?
A: GCF helps simplify fractions, solve number theory problems, and make informed decisions in real-world scenarios.

Q: What is the GCF of 0 and any other number?
A: The GCF of 0 and any number is always 0.

Greatest Common Factor Of 24 And 20

Conclusion

In the tapestry of numbers, the greatest common factor unravels the common threads that bind them together. By understanding this fundamental concept, we not only enhance our mathematical prowess but also develop problem-solving skills that reach beyond the classroom, empowering us to navigate the quantitative challenges we face daily. Whether you seek to unlock the secrets of fractions, master advanced mathematics, or simply add numerical finesse to your life, embracing the power of GCF will pave the way forward.

Is the topic of Greatest Common Factor and its applications something you’d like to explore further? Let us know in the comments below!