The concept of the greatest common factor (GCF) is fundamental in the realm of numbers, holding the power to simplify expressions, solve complex equations, and unravel intricate mathematical patterns. In this detailed odyssey, we will traverse the foundations of GCF, unearthing its significance, exploring its applications, and ultimately revealing the GCF between the quintessential numbers 6 and 9. Brace yourselves for an enlightening journey into the depths of numerical harmonies!
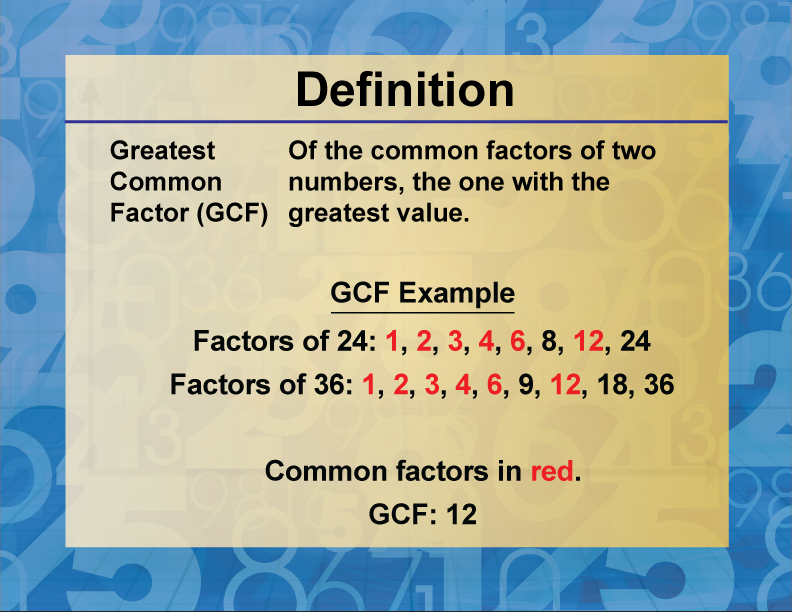
Image: www.media4math.com
Delving into the Realm of Greatest Common Factor
The greatest common factor of two or more integers denotes the largest positive integer that can be evenly divided by each of those numbers. It stands as an indication of their commonality, revealing the greatest underlying unity within their mathematical tapestry. The GCF finds remarkable utility in:
-
Simplifying Fractions: By dividing both the numerator and denominator of a fraction by their GCF, we attain its simplest form, revealing the fraction’s inherent numeracy.
-
Solving Equations: The GCF assists in the factorization of both sides of an equation, paving the way for efficient solutions. It enables us to unlock the values of variables shrouded within complex algebraic expressions.
-
Deciphering Arithmetic Patterns: The GCF unveils the core rhythm governing arithmetic sequences, empowering mathematicians to predict subsequent terms and uncover the underlying order in seemingly chaotic numerical realms.
Unveiling the GCF of 6 and 9: A Journey of Discovery
In our quest to determine the GCF of 6 and 9, we embark on a meticulous exploration of their respective prime factorizations.
6: 2 3
9: 3 3
Behold, there lies a shared elemental bond – the number 3! It gracefully emerges as the greatest common factor of 6 and 9.
Applications: Weaving Practicality into the Fabric of Concept
The GCF extends beyond its theoretical confines, unfolding an array of practical applications in our daily lives:
-
Simplifying Cooking Recipes: Scaling up or down a recipe requires adjustments to ingredient quantities. The GCF empowers us to maintain the harmonious balance of flavors by adjusting ingredients proportionally.
-
Uniting Time Zones: When coordinating schedules across different time zones, the GCF unveils the most suitable intervals at which meetings can occur for all parties involved.
-
Optimizing Train Schedules: In the intricate world of railway operations, the GCF assists in aligning train departures and arrivals to ensure seamless connections for passengers.

Image: materiallibraryflorian.z19.web.core.windows.net
What Is The Greatest Common Factor For 6 And 9
Embracing the GCF – A Continuing Mathematical Odyssey
Our journey into the realm of GCF culminates with a profound appreciation for its multifaceted nature. It transcends mere calculation; it’s a fundamental tool that unlocks numerical relationships, simplifies complexity, and illuminates the underlying patterns concealed within the enigmatic tapestry of mathematics.
As you delve deeper into the captivating world of numbers, may the GCF serve as your constant companion, guiding you through uncharted numerical territories and unraveling the secrets embedded within the harmony of numbers. Embrace the power of the greatest common factor; let it be your compass as you navigate the boundless ocean of mathematical knowledge.

