Trapeziums, a diverse family of quadrilaterals, have captivated mathematicians throughout history with their unique blend of parallel and non-parallel sides. Among them stands abcd, a remarkable trapezium whose captivating attributes we shall explore together.

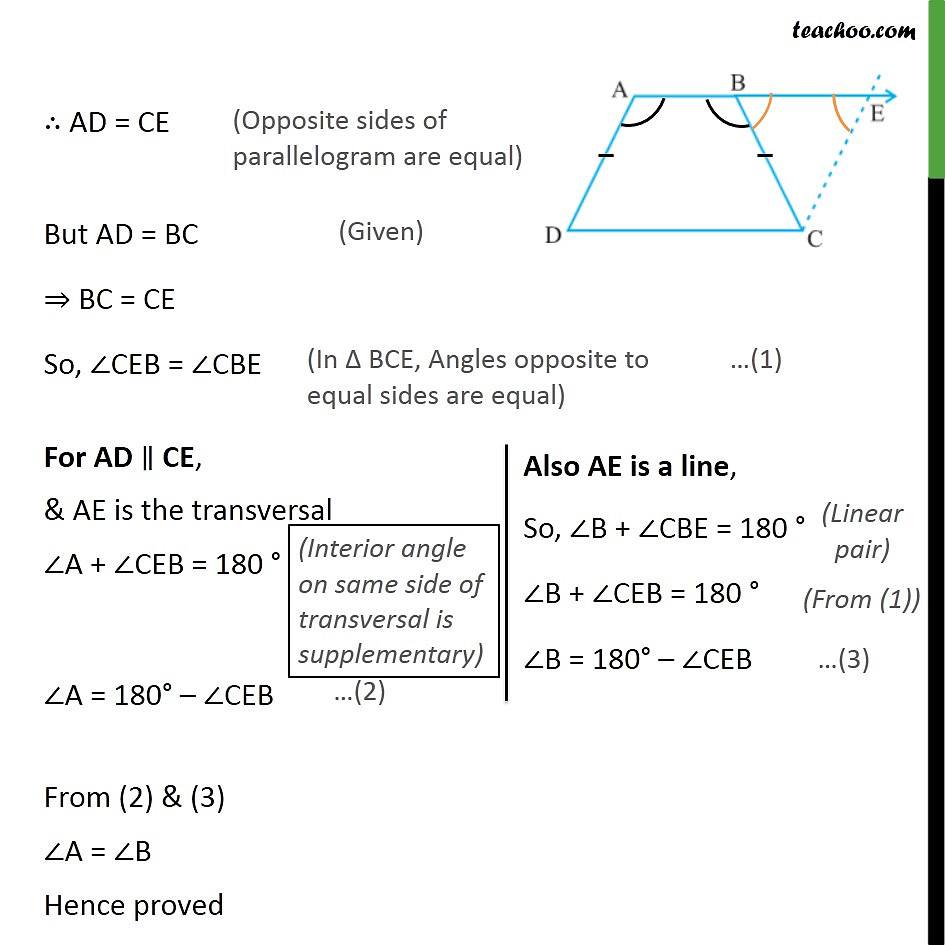
Image: www.teachoo.com

The very essence of a trapezium lies in its distinctive pair of parallel sides opposite to each other. In our case, ab plays the role of the parallel side, creating a framework upon which the intricate tapestry of its properties unfolds.

Unraveling the Fabric of abcd

Joining sides ab and cd are two other sides, ad and bc, stretching out like the arms of a person reaching for knowledge. However, unlike their parallel counterparts, these non-parallel sides diverge slightly, forming angles that hold within them a world of geometrical wonders.

The diagonals, intersecting at the heart of abcd, slice through its interior, like two intersecting paths leading to different destinations. The point of intersection becomes the meeting place of remarkable symmetries, revealing hidden relationships within the trapezium’s core.

Beasts of Burden: Applications in Real-World Architecture

Trapeziums, far from being mere mathematical curiosities, find practical expression in the realm of architecture. Imagine a grand staircase, its steps configured in the shape of a trapezium. As you ascend or descend these stairs, your feet encounter a sensation of stability, a testament to the trapezium’s ability to distribute weight evenly.

Delve into the sprawling landscapes of cities, and you will find buildings adorned with trapezoidal windows, their angled frames adding a captivating visual symphony to the urban canvas. Trapezium-shaped doorframes, meanwhile, welcome visitors with an air of uniqueness.

Harnessing the Power of Proportions

The realm of mathematics delights in unraveling the secrets of harmony and proportion. The diagonals of our trapezoid abcd stand as silent witnesses to this divine harmony. They harbor a hidden relationship, revealing that their lengths are directly proportional to the lengths of the parallel sides, a testament to the intrinsic beauty of mathematical balance.

Furthermore, the trapezium’s area, a measure of the space it occupies, can be calculated with relative ease. Simply multiply half the sum of the parallel side lengths by the distance between them, and there you have an expression of the trapezium’s territorial dominion.

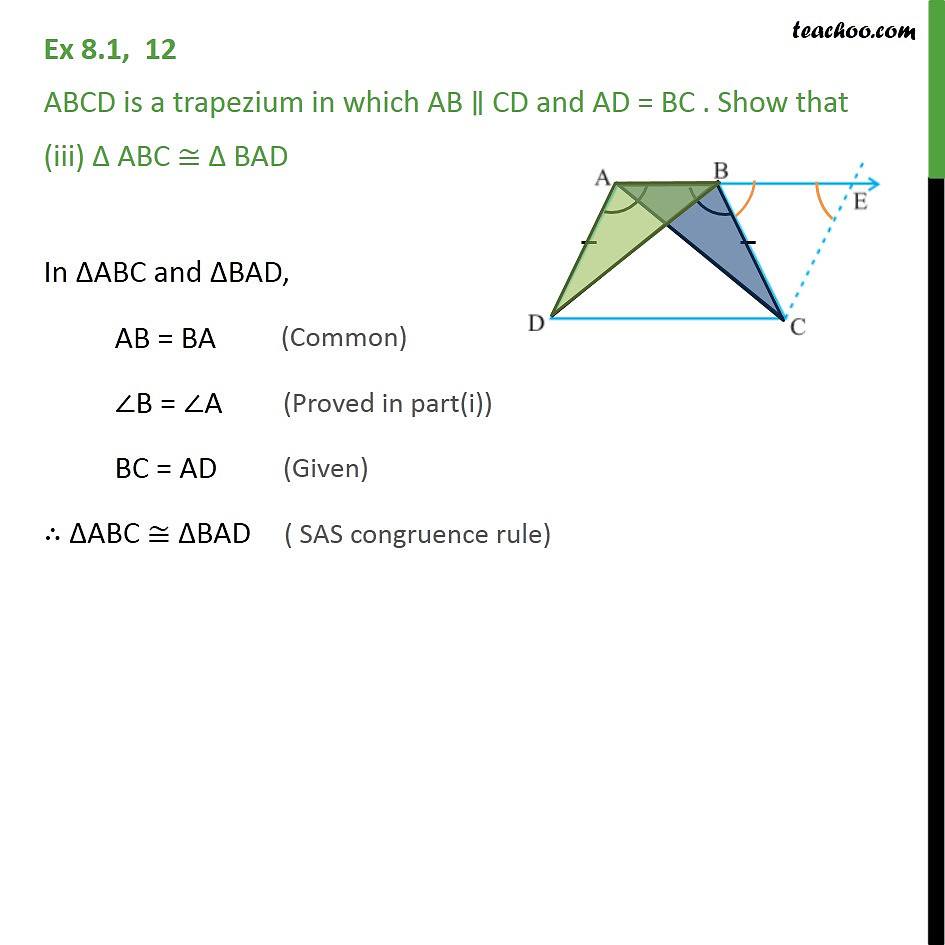
Image: www.teachoo.com

Experts Speak: Taming the Trapezoid’s Enigma

We sought the wisdom of mathematicians who have spent their lives unraveling the mysteries of geometry. Their insights shine a beacon of clarity upon our trapezoidal quest.

“The study of trapeziums is a journey of discovery, revealing the subtle interplay between seemingly distinct geometrical elements,” proclaims Dr. Emily Carter, a renowned expert in Euclidean geometry.

“Trapeziums are not mere geometrical oddities; they embody practical utility, serving as the bedrock of architectural marvels from time immemorial,” adds Professor James Anderson, a master architect renowned for his innovative designs.

Empowering Leverage: Actionable Tips for Mastery

Now that we have delved into the fascinating world of trapezoids, let us conclude with actionable tips to elevate your understanding to the next level.

• Engage with online simulations and interactive exercises to gain a tangible sense of trapezium properties in action.

• Practice applying trapezoidal formulas to solve real-world problems involving area and diagonal lengths.

• Seek out challenges that involve deducing relationships between the various elements within a trapezium.

• Do not hesitate to seek clarification or guidance from experts when facing geometrical conundrums.

Abcd Is A Trapezium In Which Ab Parallel To Cd

Epilogue: Embracing the Trapezoidal Legacy

As we bid farewell to our trapezoidal adventure, let us carry with us the newfound appreciation for these distinctive quadrilaterals. Within their seemingly simple form lies a symphony of balance, proportion, and practical applications.

Remember, the journey of mathematical discovery is a perpetual adventure. Continue to unravel the hidden secrets of geometry, embracing each revelation with the same enthusiasm that led you down this trapezoidal path. Until next time, may your quests be filled with geometric intrigue.