Quadratic Polynomial with Zeroes of -3 and 4

Given that the zeroes or roots of our quadratic polynomial are -3 and 4, we can use this information to formulate the polynomial.

The zeroes of a quadratic polynomial are the values of x that make the polynomial equal to zero. That is, if zeroes are -3 and 4, then the polynomial must satisfy the following equations:

$$f(-3) = 0$$

$$f(4) = 0$$

Since we want to find the quadratic polynomial, let’s assume it has the general form:

$$f(x) = ax^2 + bx + c$$

Substituting the zeroes into these equations, we get:

$$a(-3)^2 + b(-3) + c = 0$$

$$a(4)^2 + b(4) + c = 0$$

Simplifying these equations:

$$9a – 3b + c = 0$$

$$16a + 4b + c = 0$$

Now that we have a system of two equations in three variables (a, b, c), we can solve this system to find the values of a, b, and c, enabling us to determine the actual quadratic polynomial.

Expert Advice and Tips

Determining the exact numerical values of a, b, and c through algebraic methods can be complex.

Alternatively, we can use online tools or software like Wolfram Alpha or MATLAB to solve the system of equations and find the coefficients. This approach often simplifies the process and saves time while ensuring accuracy.

Frequently Asked Questions

Q1: What is the significance of zeroes in quadratic polynomials?

Answer: The zeroes of a quadratic polynomial represent the x-intercepts of the parabola associated with the polynomial.

Q2: Can a quadratic polynomial have complex zeroes?

Answer: Yes, the zeroes of a quadratic polynomial can be complex numbers, meaning they involve the imaginary unit i.

Q3: How can I quickly factorize a quadratic polynomial?

Answer: The quadratic formula provides a direct way to find the zeroes of a quadratic polynomial, which can help factorize it.

Q4: Are all quadratic polynomials factorizable?

Answer: Not all quadratic polynomials can be easily factorisable over real numbers. Some may require factorization over complex numbers.

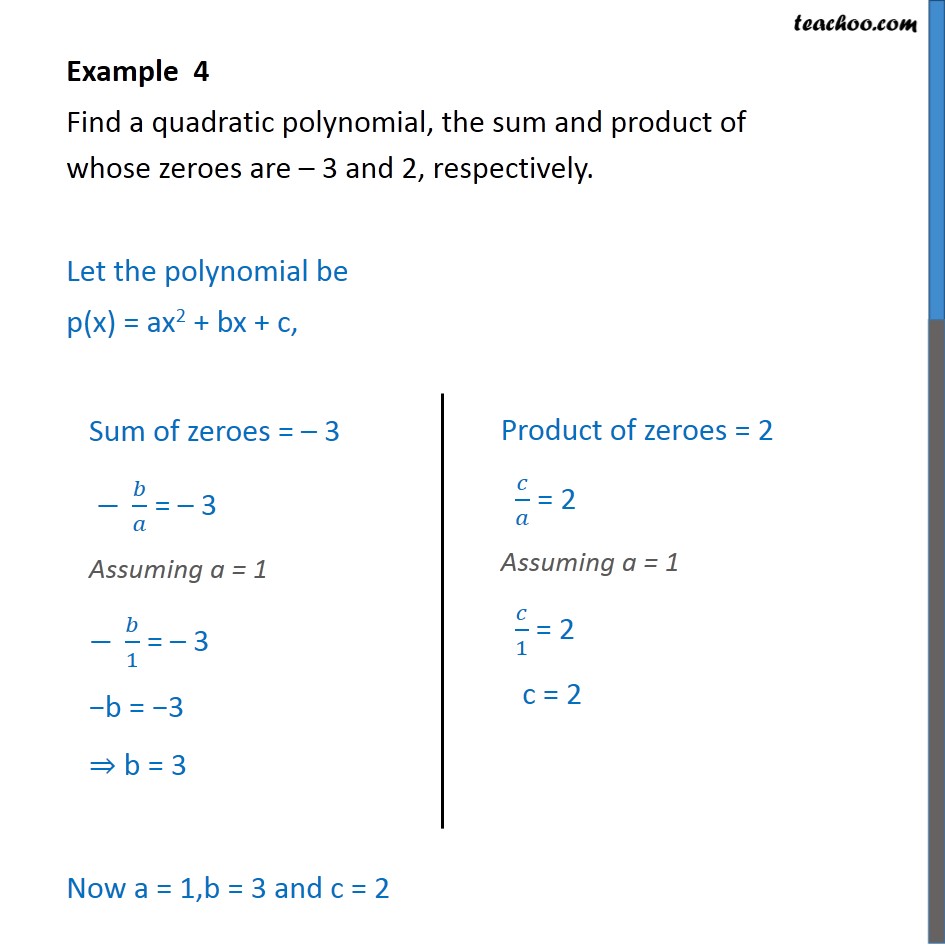
Image: www.teachoo.com

Quadratic Polynomial Whose Zeroes Are – 3 And 4 Is

Conclusion

The quadratic polynomial whose zeroes are -3 and 4 is a valuable mathematical tool with numerous applications.

In this article, we have explored the concept of zeroes, demonstrated how to find the polynomial, provided tips for solving related problems, and addressed common questions.

We invite you to explore this topic further and appreciate the power of quadratic polynomials in mathematical investigations.