Delving into triangles and proving triangle congruence

Step into the world of geometry with NCERT Class 10 Maths Chapter 6 Exercise 6.2, where we venture into the realm of proving triangle congruence. Here, we embark on an exploration of the conditions under which triangles are congruent, granting us a deeper understanding of the enigmatic world of shapes. Prepare your pencils and minds, for an adventure lies before you.

Image: www.cuemath.com

The Basics of Triangle Congruence

In mathematics, proving triangle congruence is a fundamental concept that establishes the equality of two triangles, implying that every aspect of both triangles matches precisely. When two triangles are congruent, their corresponding sides and angles coincide, enabling them to be positioned identically. This fundamental property empowers us to solve various geometric problems, uncover symmetries, and make deductions about spatial relationships.

Exploring Triangle Congruence

NCERT Class 10 Maths Chapter 6 Exercise 6.2 takes us on an in-depth journey through the intricacies of triangle congruence. Equipping ourselves with the essential tools, we embark on a step-by-step investigation of four distinct conditions that determine whether two triangles are congruent: SSS (Side, Side, Side), SAS (Side, Angle, Side), ASA (Angle, Side, Angle), and RHS (Right Angle, Hypotenuse, Side). Engaging in the study of these conditions prepares us to approach questions concerning triangle congruence with confidence and competence.

SSS Congruence: When Sides Matter

The SSS Congruence postulate serves as the cornerstone of our exploration. It asserts that if all the corresponding sides of two triangles are equal in measure, then the triangles are indeed congruent. Let’s imagine two triangles, with matching lengths for sides a, b, and c. This theorem guarantees that these triangles can be superimposed perfectly, replicating one another’s forms.

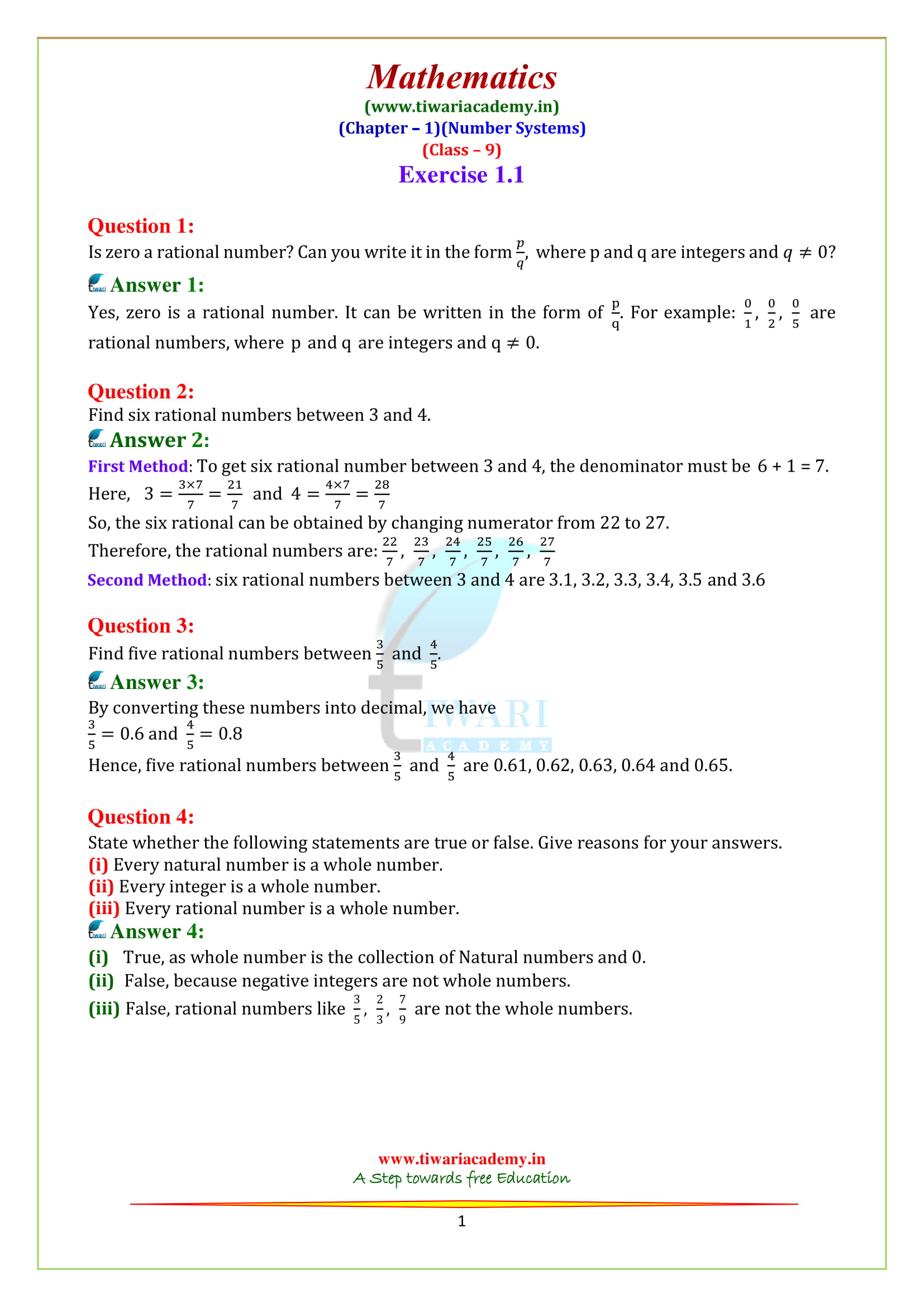
Image: www.onlinedegreeforcriminaljustice.com

SAS Congruence: Angles and Sides in Harmony

In the case of SAS Congruence, we shift our focus to the intricate relationship between sides and angles. This postulate unfolds the exciting revelation that if two pairs of corresponding sides and their intercepted angle in two triangles are congruent, then the triangles themselves are also congruent. Experience a moment of discovery as you witness these geometric connections, forging vivid mental images of these congruent triangles.

ASA Congruence: Navigating the Angle-Side-Angle Connection

We now delve into the fascinating world of ASA Congruence, where only angles play a pivotal role, in conjunction with an intervening side that adds the necessary dimension. If the corresponding angles and their non-included sides are congruent in two triangles, it’s a profound testament to their congruence. Picture these triangles gracefully overlapping, echoing each other’s every trait.

RHS Congruence: A Unique Case

Finally, our exploration of triangle congruence culminates with the intriguing case of RHS Congruence. When one pair of adjacent sides matches along with the right angles in two right-angled triangles, we can confidently declare them congruent. These geometric twins prove that congruence extends to right-angled triangles as well, reinforcing the pervasive nature of this mathematical idea.

Harnessing Triangle Congruence in Real-World Applications

Proving triangle congruence isn’t just an academic pursuit; it finds practical uses in various fields. Architects rely on congruence to create structurally sound building plans, while surveyors utilize congruence principles to map the contours of the land, ensuring precise measurements of distances and angles. Engineers harness these geometric truths to construct bridges that span vast chasms and soar to remarkable heights. The beauty of triangle congruence unravels as we discover its impact shaping the physical world around us.

Ncert Class 10 Maths Chapter 6 Exercise 6.2

https://youtube.com/watch?v=6rSdlmjdSEw

Expert Tips for Understanding Triangle Congruence

1. Visualize Triangles in Space: Sketching diagrams aids in forming a spatial understanding of triangles, fostering clarity in grasping their properties.

2. Recall the Congruence Criteria: Remembering the SSS, SAS, ASA, and RHS postulates provides critical knowledge for identifying congruent triangles.

3. Practice Problem Solving: Regularly engaging in exercises sharpens your problem-solving skills and helps you apply these criteria effectively.

4. Seek Peer Support: Collaborate with fellow learners or seek guidance from your teacher or a tutor to reinforce your understanding through discussions and questions.