As I was teaching my daughter some mathematic principles, she stumbled upon terms like complementary angles and supplementary angles.

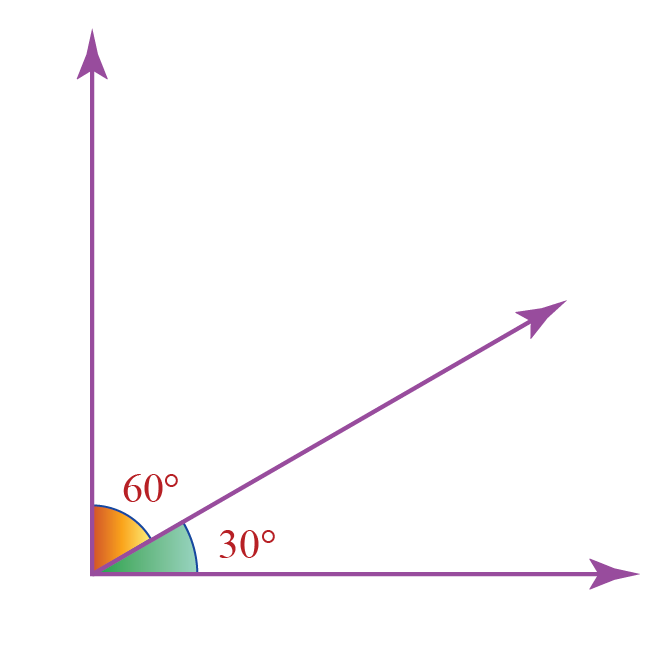
Image: www.cuemath.com

She was curious about the definitions. I was thrilled to explain these terms to my inquisitive daughter, and I realized that many students might have the same questions. So, let’s dive into the world of angles and explore these crucial concepts!

Complementary Angles

Complementary angles are a pair of angles whose measures add up to 90 degrees. They are often found when two lines intersect to form a right angle. The measure of each angle is less than 90 degrees. For example, two angles measuring 30 degrees and 60 degrees are complementary.

Properties of Complementary Angles

Complementary angles have several properties that make them unique:

• Their sum is always 90 degrees.
• They are always formed when two lines intersect to create a right angle.
• If one angle in a pair of complementary angles is known, it is easy to find the measure of the other angle by subtracting its measurement from 90 degrees.

Supplementary Angles

Supplementary angles are pairs of angles whose measurements add up to 180 degrees. They are often found when two lines intersect and form a straight line or two straight lines that are parallel and intersected by a transversal. Each angle in a pair of supplementary angles measures less than 180 degrees. For example, two angles measuring 120 degrees and 60 degrees are supplementary.

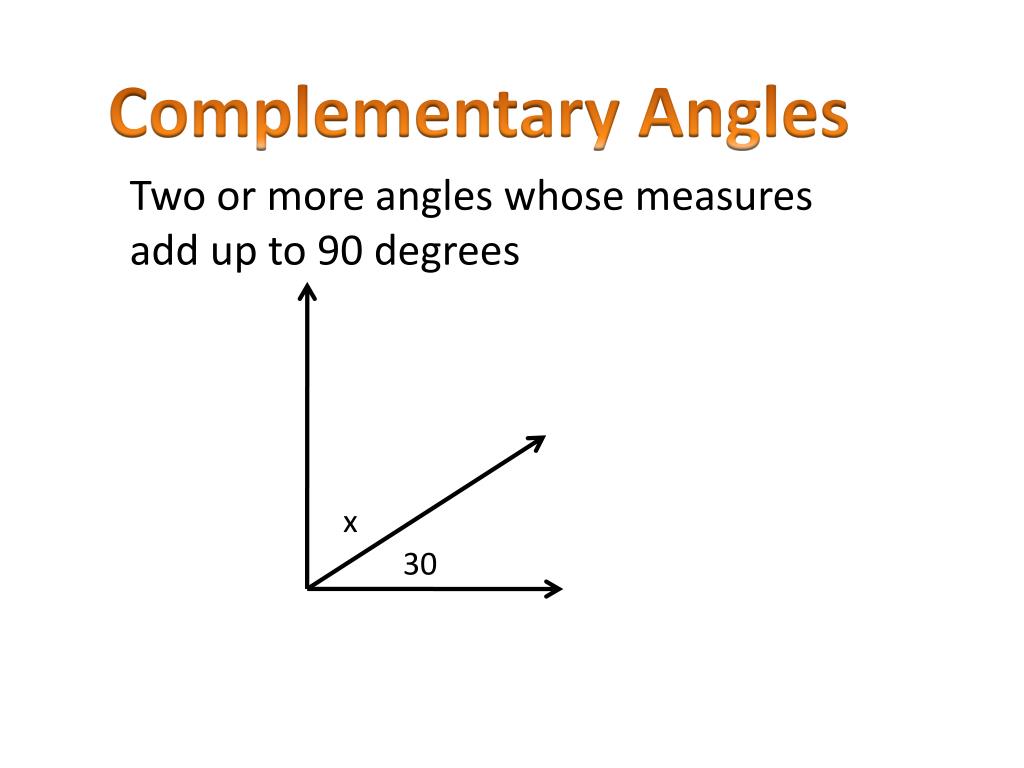
Image: www.slideserve.com

Properties of Supplementary Angles

Supplementary angles also have unique properties:

• Their sum is always 180 degrees.
• They are formed when two lines intersect to create a straight line or intersect two parallel lines which are themselves transected by another line.
• If one angle in a pair of supplementary angles is known, you can find the measure of the other angle by subtracting its measurement from 180 degrees.

Vertical Angles

In addition to complementary and supplementary angles, we have vertical angles. Vertical angles are two non-adjacent angles formed when two intersecting lines cross. These particular angles are opposite each other.

Properties of Vertical Angles

Vertical angles share important aspects:

• They are always congruent, which means they have equal measurements.
• They are formed when two lines intersect.
• The sum of the two vertical angles is always 180 degrees.

Conclusion

In geometry, angles hold a significant importance and can determine the shape and measurement of various figures. Understanding types of angles such as complementary, supplementary, and vertical angles deepens our knowledge about angles and their significance in geometry and everyday life.

If you’re interested in further exploring these concepts or have any specific questions, reach out and dive deeper into the captivating world of angles!

What Is The Sum Of Complementary Angles

FAQs on Angles

Q: What’s perpendicular mean?

A: Two lines or angles are perpendicular when they intersect, forming a right or 90-degree angle.

Q: What is the angle bisector of a vertical angle?

A: An angle bisector is a ray that divides an angle into two equal angles. In the case of a vertical angle, the bisector is a line that passes through the vertex of the angle and is perpendicular to the lines forming the vertical angle, effectively creating two congruent right angles.

Q: Can an obtuse angle be part of a pair of complementary angles?

A: No, obtuse angles cannot be part of complementary angles. Complementary angles must add up to 90 degrees, and obtuse angles measure greater than 90 degrees.

Q: How can you find the missing angle in a situation involving a pair of supplementary angles?

A: Assume the measure of one angle as “x”. Since supplementary angles have a sum of 180 degrees, the other angle must measure “180 – x”. You can leverage this to solve for the missing measurement.