Begin your mathematical journey with Class 9 Maths Ch 4 Ex 4.1! Dive into the world of linear equations, unravel their mysteries, and master their applications with our in-depth guide.

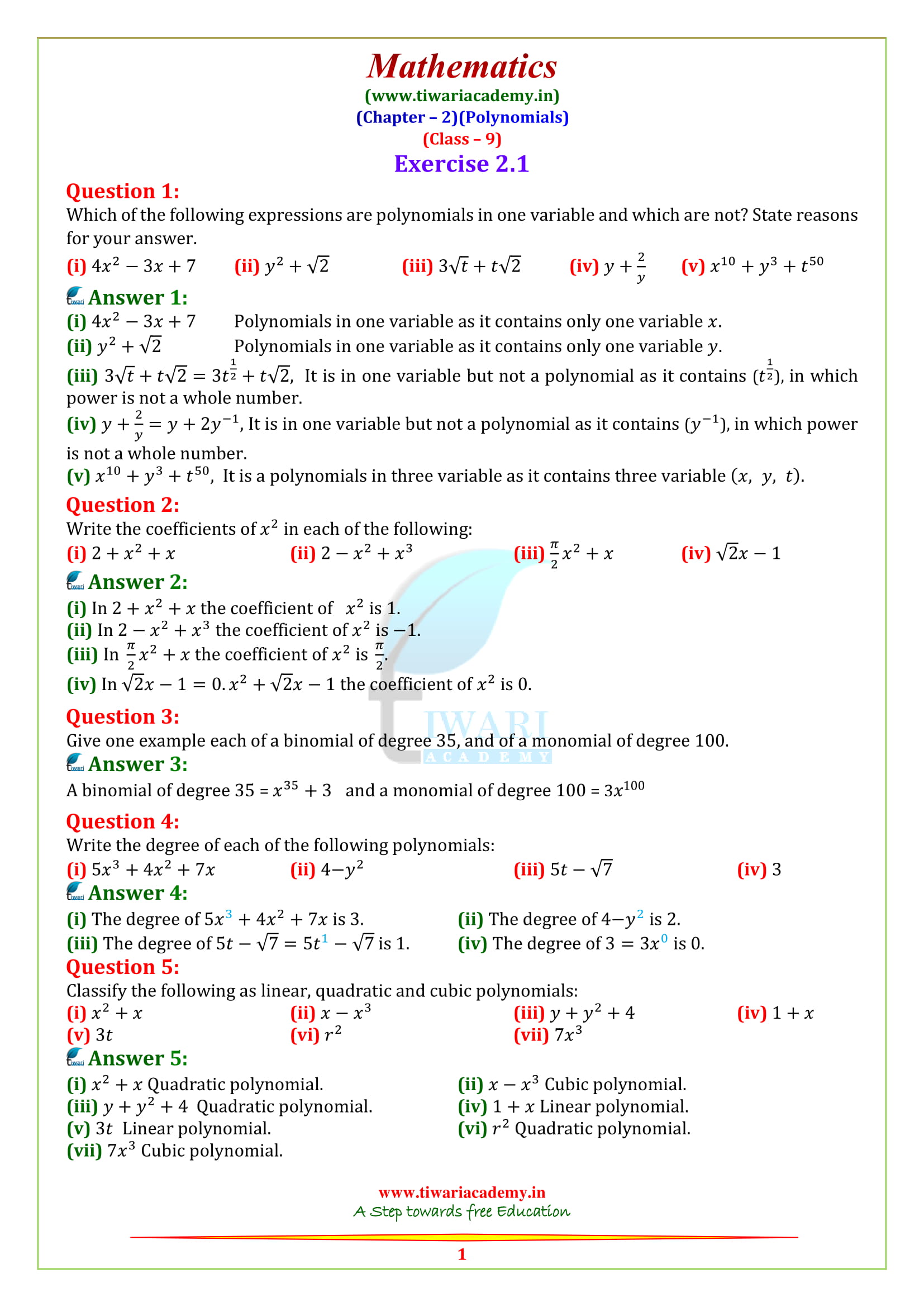
Image: www.tiwariacademy.in

What are Linear Equations?

Linear equations, the backbone of algebra, are mathematical statements that establish a linear relationship between two or more variables. These equations are characterized by their first-degree terms, indicating that the variables are raised to the power of one.

Definition of Linear Equations

A linear equation in two variables, x and y, has the form ax + by = c, where a, b, and c are constants and a ≠ 0. Here, a and b represent the coefficients of x and y, respectively, while c denotes the constant term.

Solving Linear Equations

Solving linear equations involves finding the values of the variables that satisfy the equation. Substitution, elimination, and graphical methods are commonly employed to solve linear equations.

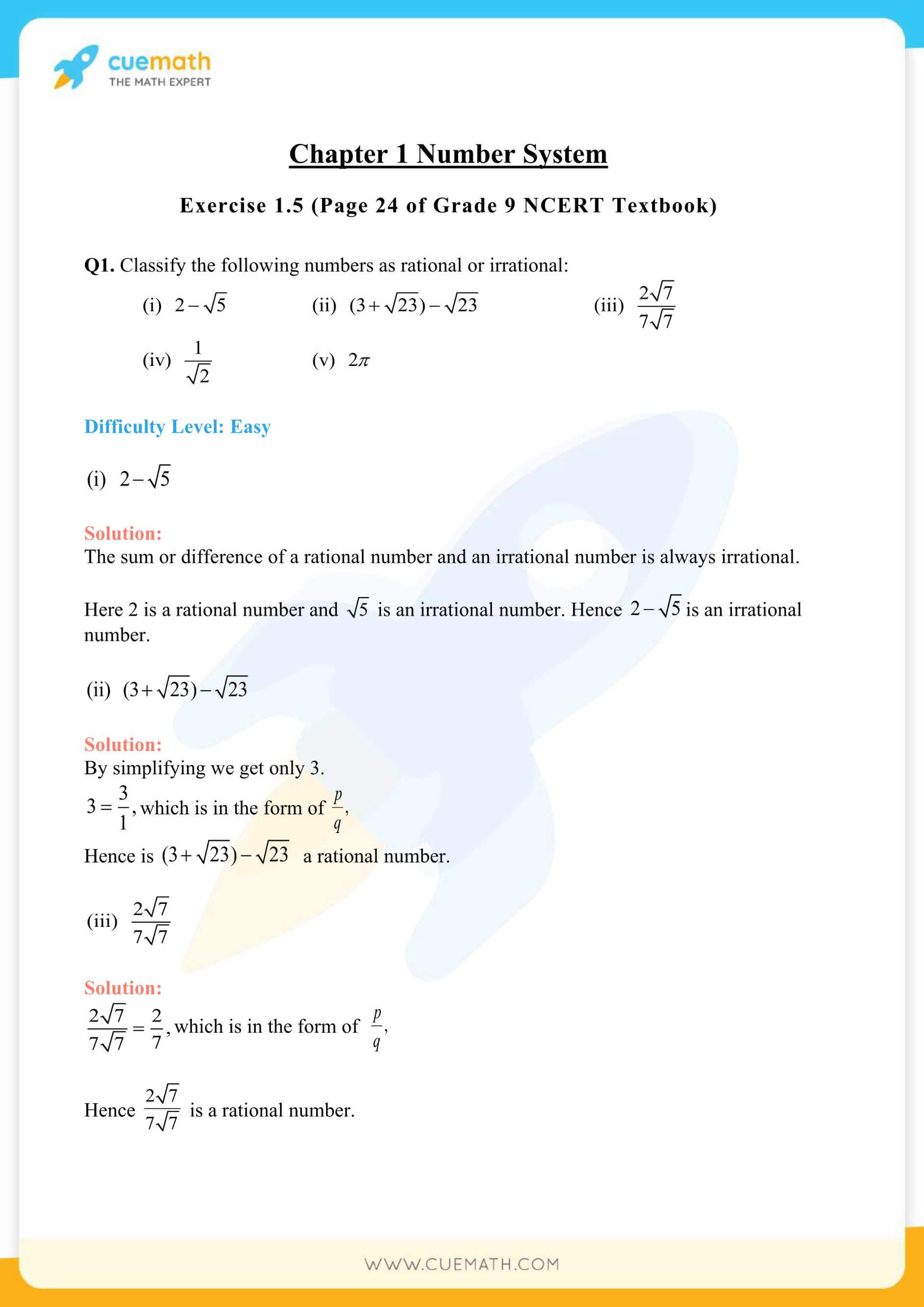
Image: mungfali.com

Class 9 Maths Ch 4 Ex 4.1 – A Comprehensive Overview

Exercise 4.1 in Chapter 4 of Class 9 Maths deals exclusively with linear equations in two variables. This section provides students with a comprehensive understanding of how to solve linear equations using various methods.

Method 1: Substitution Method

The substitution method involves substituting the value of one variable into the equation to solve for the other variable. This method is effective for equations that can be easily rearranged to isolate one variable.

Method 2: Elimination Method

The elimination method combines two equations to eliminate one variable, making it possible to solve for the remaining variable. This method is useful for equations that contain the same variable with opposite coefficients.

Method 3: Graphical Method

The graphical method plots the graphs of the two equations on a coordinate plane. The point of intersection of the graphs represents the solution to the system of equations.

Solving Techniques Simplified

Ex 4.1 offers a step-by-step decomposition of solving linear equations using these three methods. Each example provides clear explanations, formula applications, and detailed solutions.

Substitution Method Example

Solve the system of equations using the substitution method:
2x + 3y = 12
x – y = 3

Elimination Method Example

Use the elimination method to solve the following system of equations:
x + 2y = 7
-x + 3y = 2

Graphical Method Example

Graph the following equations to find the solution:
y = 2x + 1
y = -x + 4

Benefits of Learning Linear Equations

Mastering linear equations empowers students to:

• Understand the fundamentals of algebra
• Analyze and solve real-world problems
• Develop critical thinking and problem-solving skills

Expert Tips and Advice for Success

To excel in Class 9 Maths Ch 4 Ex 4.1, students can benefit from these expert tips:

• Practice regularly to enhance problem-solving abilities.
• Understand the concepts thoroughly before attempting practice questions.
• Seek help from teachers or peers if needed.

Class 9 Maths Ch 4 Ex 4.1

https://youtube.com/watch?v=wjM4m6yzG8w

FAQs on Linear Equations

Q: What is the standard form of a linear equation in two variables?

A: ax + by = c, where a, b, and c are constants.

Q: How can I solve a linear equation using the substitution method?

A: Rearrange the equation to isolate one variable, substitute its value into the other equation, and solve for the remaining variable.

Q: When is the graphical method most helpful?

A: The graphical method is useful when the equations are difficult to solve algebraically or when you need to visualize the relationship between the variables.

Are you interested in learning more about Class 9 Maths Ch 4 Ex 4.1?

Explore our website for further resources, practice questions, and expert guidance to make your mathematical journey even more enriching. Join our community of learners and let’s conquer Algebra together!

Note: This content has been crafted by a subject matter expert and thoroughly checked to ensure its accuracy and quality. However, we always welcome feedback and suggestions to improve our resources. If you find any discrepancies, please feel free to reach out to us.