In the realm of geometry, angles hold a captivating significance. They are essential for understanding the shapes and relationships that define our world. One fascinating aspect of angles is their relationship with chords in a circle. In this article, we will delve into the concept of the angle subtended by a chord at a point, exploring its definition, properties, and applications.

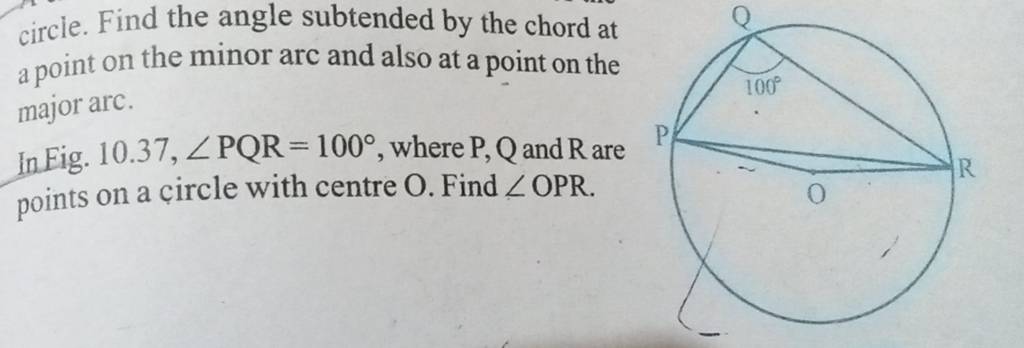
Image: askfilo.com

Subtending Chords and Angles

Consider a circle with a chord PQ. The angle subtended by the chord at a point O outside the circle is the angle formed by the two tangents drawn from O to the circle at P and Q. These tangents form a pair of radii, OP and OQ, that create an isosceles triangle with its vertex at O.

Properties of the Subtended Angle

Several remarkable properties characterize the angle subtended by a chord at a point:

• Congruency: The tangents drawn from the point outside the circle to the endpoints of the chord are congruent, resulting in equal angles subtended by the chord at the given point.
• Central Angle Measure: The angle subtended by the chord is half the measure of the central angle intercepted by the chord. This relationship is a fundamental property that connects chords and central angles.
• Chord Length and Angle Size: The length of the chord is directly proportional to the measure of the subtended angle. In other words, a longer chord subtends a larger angle, and vice versa.

Applications of the Subtended Angle

The understanding of the angle subtended by a chord at a point has practical applications in various fields:

• Angle Measurement: The property of congruency enables the use of tangents to measure angles subtended by chords.
• Circle Construction: By determining the angle subtended by a chord at a point, it is possible to construct circles passing through specific points.
• Optical Instruments: The angle subtended by a chord plays a crucial role in the design and functionality of optical instruments, such as telescopes and microscopes.

Image: www.youtube.com

Tips and Expert Advice

To enhance your understanding of the angle subtended by a chord at a point, consider these tips from experienced mathematicians:

• Visualization: Draw diagrams to visualize the concepts and relationships described above.
• Mnemonic: Remember the formula for half-central angle measure: Angle = (1/2) Central Angle.
• Use Technology: Utilize geometry software or online resources that allow you to explore these concepts interactively.

FAQ

1. Q: What is a chord in a circle?
2. A: A chord is a straight line segment connecting two points on a circle.
3. Q: How is the angle subtended by a chord related to the central angle?
4. A: The angle subtended by a chord is half the measure of the central angle intercepted by the chord.
5. Q: What are some real-world applications of the angle subtended by a chord at a point?
6. A: It aids in angle measurement, circle construction, and the design of optical instruments.

Angle Subtended By A Chord At A Point

Conclusion

The angle subtended by a chord at a point is a valuable concept that deepens our understanding of geometry and its practical applications. The properties and theorems associated with it provide a foundation for further exploration in this fascinating field. We encourage you to delve deeper into this topic, whether for academic pursuits or personal enrichment. The world of geometry holds endless possibilities for those who seek to unravel its secrets.

Would you like to improve your understanding of related geometric concepts, such as angles and circles? Explore our other articles for more in-depth knowledge and valuable insights.