Mathematics, a subject that often evokes a mix of excitement and trepidation among students, becomes particularly fascinating when we venture into the realm of geometry. Class 9 Maths Chapter 6 Ex 6.1 introduces us to the fundamental concepts of lines and angles, laying a cornerstone for our understanding of more complex geometric structures in the future.
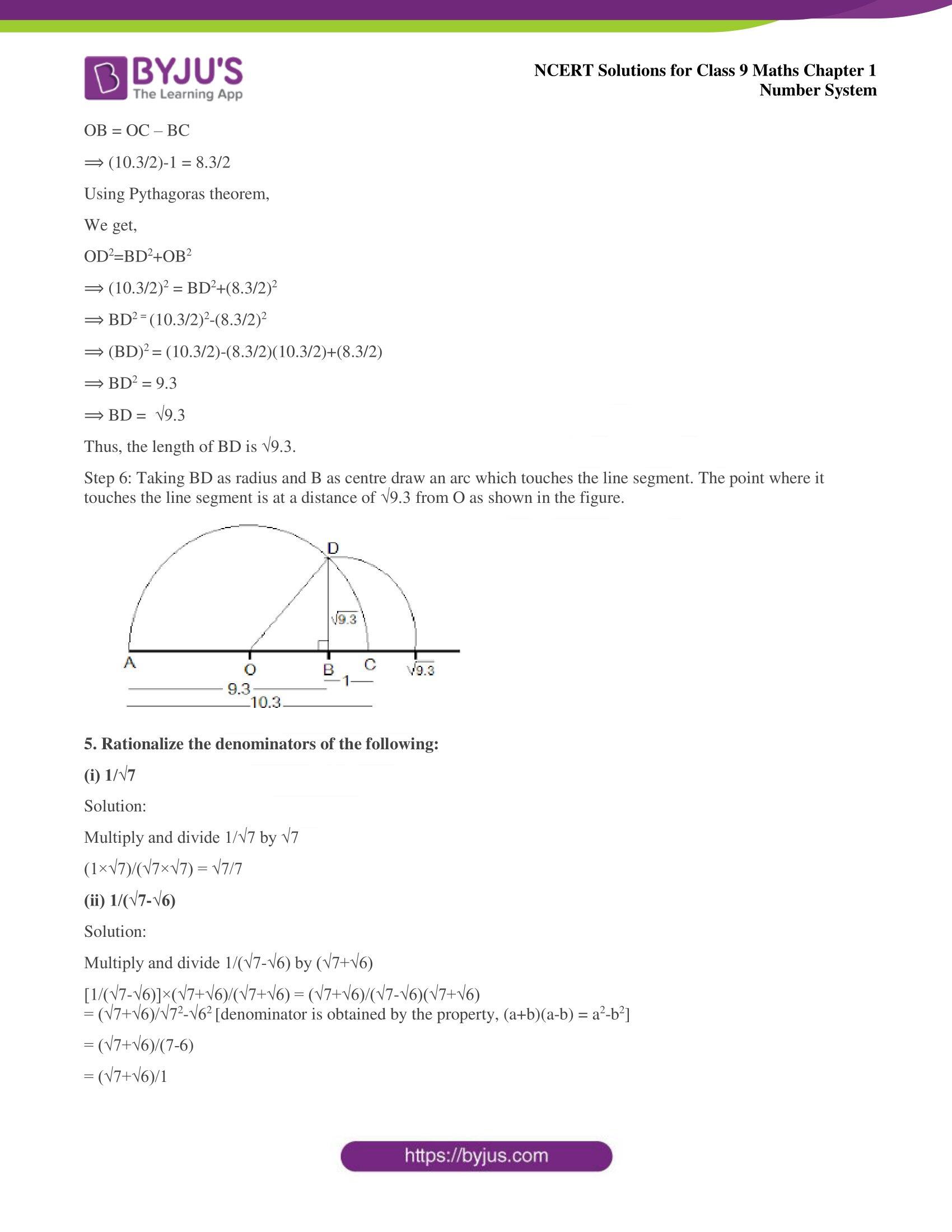
Image: byjus.com
Geometry, derived from the Greek word “geo” meaning “earth,” has played a pivotal role in shaping human civilization. From the construction of pyramids to the design of intricate architectural marvels, geometry has been an indispensable tool in engineering, navigation, and even art.
The Beauty of Lines and Angles
Definition of a Line
In mathematics, a line is defined as a one-dimensional figure that extends infinitely in both directions. It has no width or height and is represented by an arrow to indicate its direction.
Lines can be classified into different types based on their relationship to each other. Parallel lines are lines that never intersect, intersecting lines are lines that cross at a single point, and perpendicular lines are intersecting lines that form a 90-degree angle at their point of intersection.
Definition of an Angle
An angle is formed when two rays (half-lines) share a common endpoint. The point where the rays meet is called the vertex of the angle. The angle is measured in degrees, using units of arc. A complete rotation is equivalent to 360 degrees.
Angles can be classified into different types based on their measure. Acute angles are angles less than 90 degrees, obtuse angles are angles greater than 90 degrees, right angles are exactly 90 degrees, and reflex angles are angles greater than 180 degrees but less than 360 degrees.
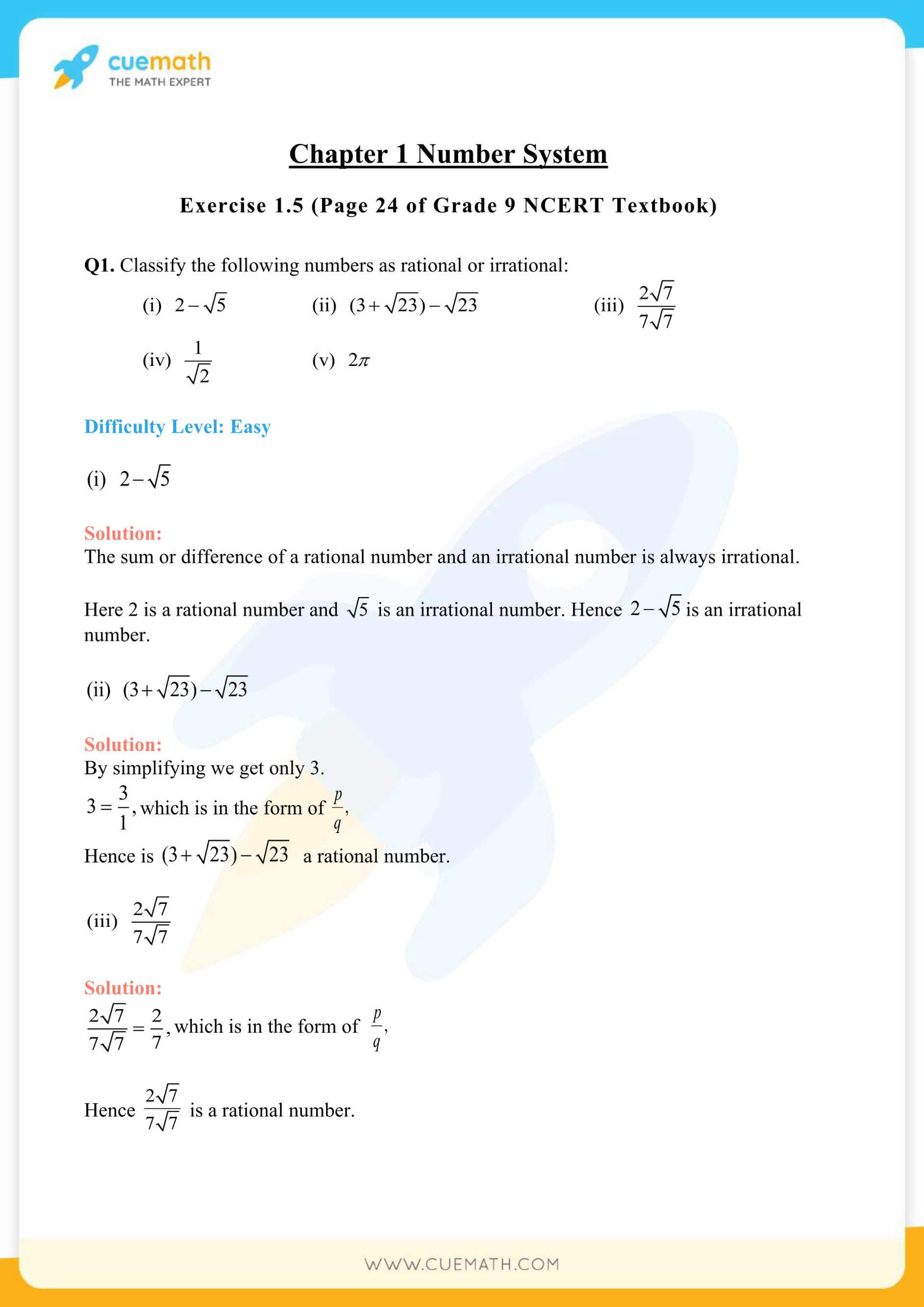
Image: printablezonemoore.z19.web.core.windows.net
Real-World Applications of Lines and Angles
The concepts of lines and angles find numerous applications in various fields, including architecture, engineering, and design.
- Architects use lines and angles to create blueprints and structural plans, ensuring the stability and aesthetic appeal of buildings.
- Engineers rely on lines and angles to design bridges, roads, and other infrastructure, ensuring their functionality and durability.
- Designers utilize lines and angles to create visually appealing products and user interfaces, enhancing both form and function.
Mastering Class 9 Maths Chapter 6 Ex 6.1
To excel in Class 9 Maths Chapter 6 Ex 6.1, a thorough understanding of the underlying concepts is crucial. Here are some tips and expert advice to help you succeed:
1. Understand the Definitions: Ensure that you have a clear understanding of the definitions of lines and angles before proceeding with the exercises.
2. Practice Identifying and Classifying: Practice identifying and classifying different types of lines and angles to strengthen your understanding of their characteristics.
3. Study Theorems and Properties: Study the various theorems and properties related to lines and angles, as they will serve as a foundation for solving more complex problems.
4. Utilize Diagrams: Draw diagrams whenever necessary to visualize the concepts and facilitate better understanding.
5. Stay Consistent: Consistency is key when it comes to learning mathematics. Dedicate regular time to practice and reinforce your knowledge.
FAQs on Class 9 Maths Chapter 6 Ex 6.1
- Q: What is the difference between a line and a ray?
A: A line extends infinitely in both directions, while a ray extends infinitely in only one direction. - Q: How do you measure an angle?
A: Angles are measured in degrees using an angle-measuring device called a protractor. - Q: What is the sum of the angles in a triangle?
A: The sum of the angles in any triangle is always 180 degrees. - Q: What is the difference between an acute and an obtuse angle?
A: An acute angle is less than 90 degrees, while an obtuse angle is greater than 90 degrees. - Q: What is perpendicular bisector?
A: A perpendicular bisector is a line that passes through the midpoint of a line segment and is perpendicular to the line segment.
Class 9 Maths Ch 6 Ex 6.1
Conclusion
Class 9 Maths Chapter 6 Ex 6.1 provides an insightful introduction to the fundamentals of lines and angles. By mastering the concepts and following the expert advice outlined in this article, students can build a

