As a mathematics enthusiast, I’ve often delved into the captivating world of prime factorization, the art of breaking down numbers into their prime building blocks. One such number that has intrigued me is 841. Join me as we embark on a mathematical expedition to uncover the square root of 841 through the lens of prime factorization, revealing its intricate numerical story.
Image: mathmonks.com
Diving into Prime Factorization: Breaking Down 841
Prime factorization, a cornerstone of number theory, involves decomposing positive integers into the product of their prime number factors. A prime number is a whole number greater than 1 that cannot be expressed as a product of two smaller whole numbers. Primes are the fundamental building blocks of all integers, including the intriguing number 841.
To determine the prime factorization of 841, we embark on a methodical process of repeatedly dividing the number by prime numbers until we are left with 1. Let’s trace this process:
- 841 ÷ 29 = 29 (Prime, division stops)
Thus, we uncover that 29 is a prime factor of 841.
- 29 ÷ 29 = 1 (No division, factorization complete)
With no further prime decomposition possible, we conclude that prime factorization of 841 indeed yields a single prime factor: 29.
Delving into the Square Root of 841
Now that we have dissected 841 into its prime factors, we can explore the extraction of its square root. The square root of a number is the value that, when multiplied by itself, results in the original number.
Since 841 has only one prime factor, 29, we can directly determine its square root:
√841 = √(29^2)
= 29
Hence, the square root of 841 is 29.
Latest Trends and Developments in Prime Factorization
Prime factorization continues to play a vital role in modern mathematics and computer science. As algorithms become increasingly sophisticated, prime factorization techniques are being applied to complex problems such as:
- Cryptography: Encoding and decoding messages securely
- Integer factorization: Solving complex mathematical challenges
- Artificial intelligence: Optimizing machine learning models
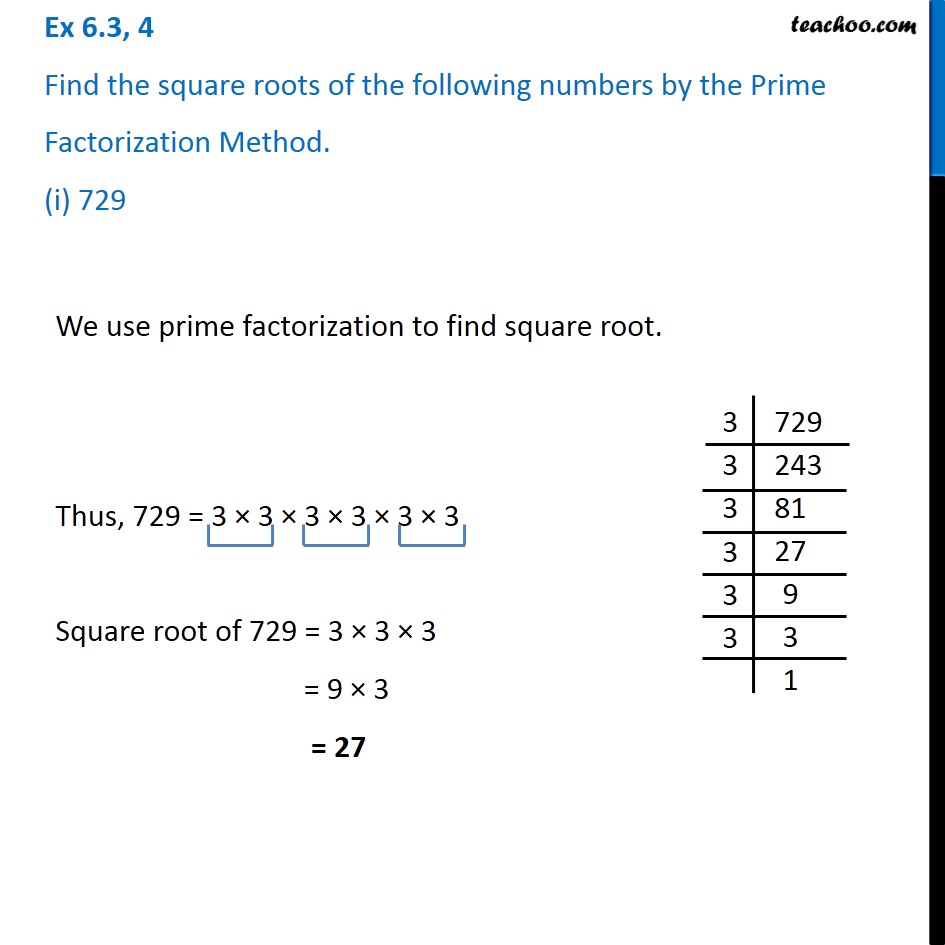
Image: www.teachoo.com
Tips and Expert Advice for Prime Factorization
Mastering prime factorization requires practice and an intuitive grasp of mathematical principles. Here are some expert tips to enhance your understanding:
- Familiarize yourself with prime numbers: Delve into their patterns and properties, recognizing their uniqueness.
- Practice factor trees: Create visual representations of prime factorizations, branching out from the number like a tree.
- Utilize prime factorization algorithms: Investigate techniques like the Fermat method or trial division for efficient factorization.
By incorporating these tips into your learning journey, you will gain a solid foundation in prime factorization.
FAQs on Prime Factorization
Q: Why is prime factorization important?
A: Prime factorization is crucial for understanding the structure of numbers, solving mathematical problems, and underpinning cryptographic algorithms.
Q: How do I find all prime factors of a number?
A: Systematically divide the number by increasingly larger prime numbers until reaching 1, recording each prime factor encountered.
Q: What are some applications of prime factorization?
A: Prime factorization finds widespread use in cryptography, computer science, number theory, and various scientific disciplines.
Square Root Of 841 By Prime Factorization
Conclusion: Unlocking the Mathematical Treasures
Our exploration of the square root of 841 through prime factorization has uncovered the elegance and simplicity of this mathematical operation. By understanding the prime factors of a number, we gain deeper insights into its numerical essence. Whether you are a seasoned mathematician or an inquisitive mind eager to unlock the secrets of prime factorization, I invite you to delve further into this fascinating subject. Its beauty and practical applications continue to captivate and inspire generations of learners. Let us know if you have any further queries or wish to delve deeper into the wonders of prime factorization.

