In the vast realm of mathematics, sequences play a pivotal role, paving the way for solving diverse problems and unraveling intricate patterns. Amidst the myriad types of sequences, arithmetic sequences stand out, intriguing the minds of learners for centuries. If you’re on a quest to master arithmetic sequences and decipher the elusive first term, this guide will illuminate the path to mathematical enlightenment.

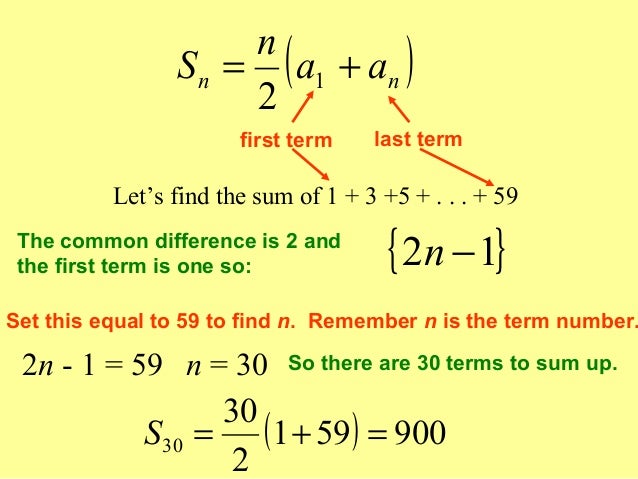
Image: www.slideshare.net

1. Decoding the Essence of Arithmetic Sequences

Imagine a sequence of numbers, like the rhythmic beats of a drummer, where each successive term faithfully follows a constant difference from its predecessor. Behold, this harmonious progression is the very nature of an arithmetic sequence. In essence, the difference between any two consecutive terms remains unwavering, much like the unwavering tempo of a metronome.

2. Establishing a Notational Framework

To embark on our mathematical excursion, let us unfurl the notation that governs arithmetic sequences. Let a₁, a₂, a₃, …, an represent the enchanting dance of numbers. Here, a₁ signifies the first term—the pivotal element we seek to unearth. The elusive constant difference that orchestrates this rhythmic progression is aptly denoted by ‘d’.

3. A Formulaic Gateway: Unveiling the First Term

With notation as our compass, we embark on the quest to unravel the formula that will unveil the first term:

a₁ = a – (n –1)d

Let’s illuminate the intricacies of this formula:

a: Denotes an arbitrary term in the sequence
n: Indicates the position of the term we seek
d: Represents the steadfast difference between terms

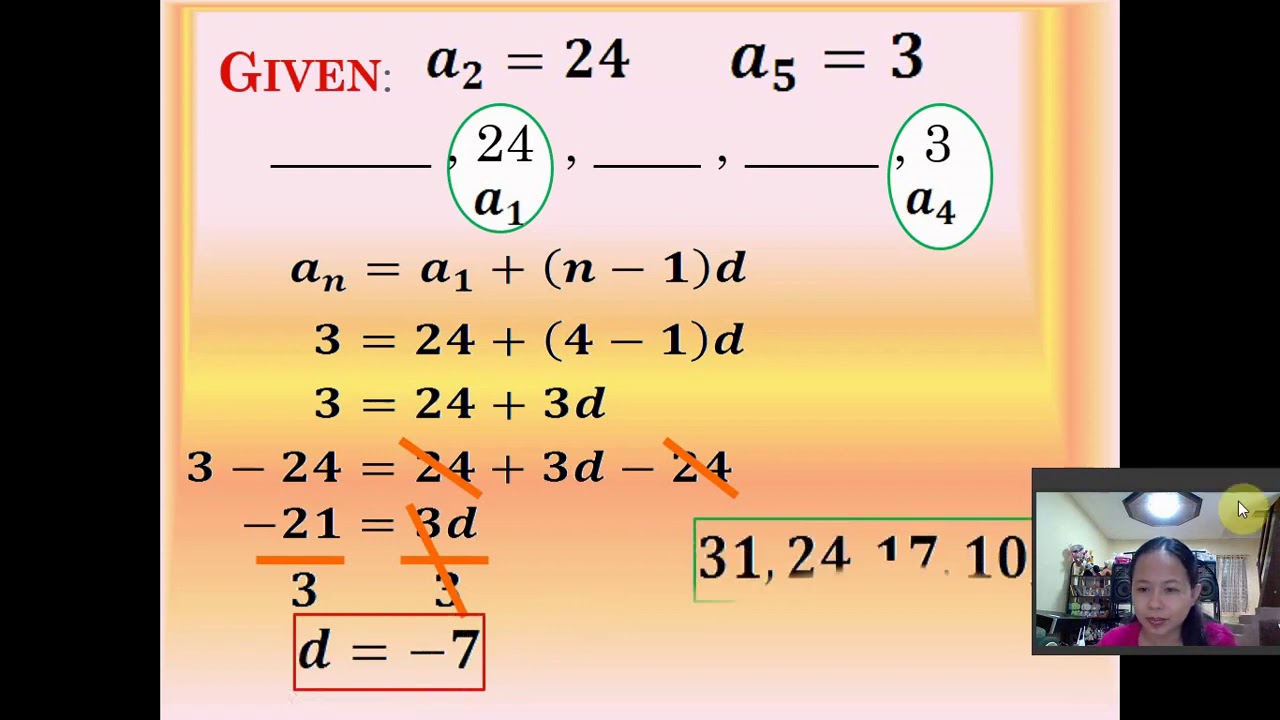
Image: www.youtube.com

4. Deconstructing the Formula: Unveiling Its Inner Workings

To comprehend the formula’s elegance, let’s dissect it step by step:

a – (n–1)d = a –(nd – d) = a – nd + d

The interplay of terms reveals a fascinating pattern. The mysterious first term, a₁, can be obtained by deducting (n-1) times the difference ‘d’ from an arbitrary term ‘a’. This numerical dance unravels the secret behind finding the first term.

5. A Practical Example: Illuminating the Path

Let’s embark on a practical journey to cement our understanding. Consider a captivating arithmetic sequence: 5, 8, 11, 14, 17, …

a = 11 (an arbitrary term)
n = 3 (position of the chosen term)
d = 3 (constant difference)

Plugging these values into our formula, we embark on a mathematical adventure:

a₁ = 11 – (3–1)3 = 11 – 2 × 3= 5

Eureka! We’ve unearthed the first term of the sequence: a₁ = 5. The harmonious pattern unravels before our eyes, each term diligently adhering to the constant difference of 3.

6. Beyond the Formula: Exploring the Experts’ Insights

To enrich our understanding, let’s delve into the wisdom of renowned mathematicians who have graced the field of arithmetic sequences.

Pythagoras, a revered figure in the annals of mathematics, famously stated, “Number rules the universe.” His words resonate with the rhythmic nature of arithmetic sequences, where numbers dance in perfect harmony.

Leonardo Fibonacci, the brilliant Italian mathematician, observed, “The sum of the first n terms of an arithmetic sequence is equal to n/2 (first term + last term).” This profound insight unravels the intricate relationship between the first term and the overall sum of an arithmetic sequence.

7. A Wealth of Applications: Where Arithmetic Sequences Thrive

The practical applications of arithmetic sequences extend far beyond the confines of textbooks. From the mundane to the magnificent, they permeate our lives in countless ways:

• Finance: Calculating interest payments, loan repayments, and investment returns.
• Physics: Describing the motion of objects under constant acceleration.
• Engineering: Designing structures to withstand varying loads and stresses.

8. A Clarifying Interlude: Dispelling Common Misconceptions

As we navigate the realm of arithmetic sequences, certain misconceptions may cloud our understanding. Let us dispel these illusions:

• Myth: All sequences are arithmetic sequences.
  Fact: Arithmetic sequences are a specific type of sequence characterized by a constant difference between terms.

• Myth: The difference between consecutive terms in an arithmetic sequence is always a whole number.
  Fact: While the difference is often a whole number, it can also be a fraction or even an irrational number.

How To Find The First Term Of An Arithmetic Sequence

9. Nurturing Mathematical Proficiency: Embracing Challenges

To solidify your grasp of arithmetic sequences, engage in thoughtful practice. Solve problems, challenge yourself with riddles, and seek guidance from mentors and resources. Remember, perseverance is the key to unlocking mathematical mastery.

Conclusion: The First Term Unveiled, A Journey Completed

Through this in-depth exposition, we’ve illuminated the enigmatic world of arithmetic sequences. We’ve unearthed the formula for finding the first term, explored expert insights, and delved into practical applications. As you continue your mathematical journey, remember that the pursuit of knowledge is an unending adventure, where each discovery unlocks new horizons.