Introduction
In the realm of mathematics, the concept of variation lies at the heart of understanding the relationships between variables. This intricate concept is particularly significant in fields like science and economics, where understanding how one quantity changes in response to another is quintessential to problem-solving and effective decision-making.
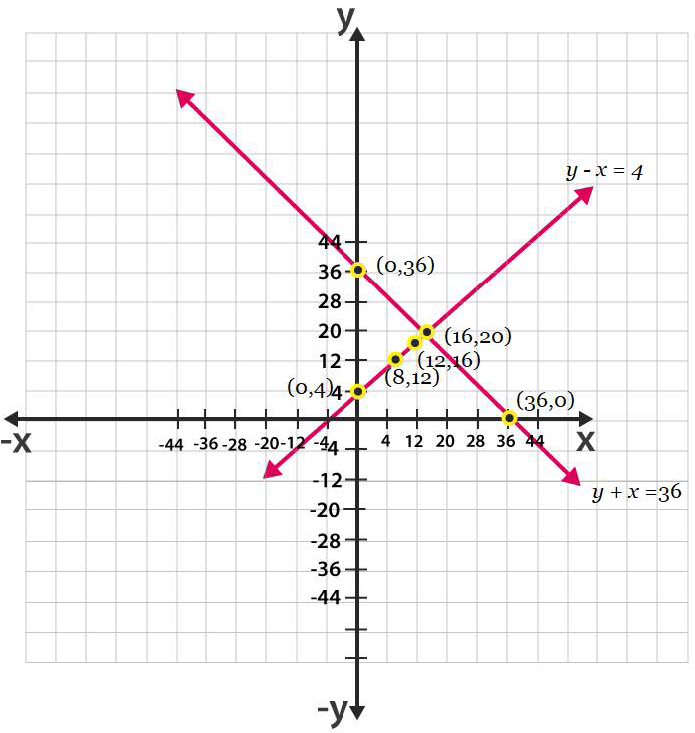
Image: itselfubseb10.blogspot.com
In this comprehensive guide, we delve into two fundamental types of variations – direct variation and inverse variation. We will explore their definitions, real-world applications, and techniques to solve related problems in mathematics.
Direct Variation
In a direct variation relationship, two variables are directly proportional to each other. As one variable increases, the other variable proportionally increases as well. A classic example is the relationship between distance and time when traveling at a constant speed. As you travel for a longer period (time), you cover a greater distance. Mathematically, direct variation can be represented as:
y = kx
where:
- y represents the dependent variable
- x represents the independent variable
- k is a constant of proportionality
Applications of Direct Variation
Direct variation has a myriad of applications in various fields. Some common examples include:
-
Physics: Force exerted by an object directly varies with its mass when kept at a constant gravitational field (F = ma).
-
Economics: The cost of producing goods often varies directly with the number of units being produced.
Inverse Variation
In an inverse variation relationship, two variables are indirectly proportional to each other. As one variable increases, the other variable proportionally decreases. A simple example is the relationship between time taken to complete a task and the number of people working on it. As the number of people increases, the time taken to complete the task decreases, assuming all work at the same rate. Mathematically, inverse variation is expressed as:
y = k/x
where:
- y represents the dependent variable
- x represents the independent variable
- k is a constant of proportionality
Image: uniquestudyonline.com
Applications of Inverse Variation
Inverse variation finds applications in various scenarios:
-
Science: The speed of a moving object varies inversely with the resistance force (v = k/r).
-
Economics: The supply of a product may be inversely related to its price.
Problem-Solving Techniques
Solving problems involving direct and inverse variations requires a few simple steps:
-
Identify the Relationship: Determine if the relationship is direct or inverse variation based on the problem description or the given equation.
-
Determine the Constant of Proportionality: Find the value of k using the given information or by examining data points in the problem.
-
Solve for the Unknown Variable: Using the substitution method or other algebraic techniques, isolate and solve for the unknown variable.
Examples
- Direct Variation: If a car travels at a constant speed of 60 mph, the distance covered (y) is directly proportional to the time (x) spent traveling. Determine the distance covered in 3 hours:
y = kx
y = 60x
y = 60(3)
y = 180 miles
- Inverse Variation: A small business observed that the time (y) taken to produce a certain number of items is inversely proportional to the number of workers (x). It takes 10 hours when completed by 5 workers. How much time would it take if the task is completed by 8 workers?
y = k/x
10 = k/5
k = 50
y = 50/x
y = 50/8
y = 6.25 hours
Class 9 Ch 13 Maths Ex 13.1
Conclusion
Understanding the concepts of direct and inverse variations is essential for tackling a wide range of mathematical problems related to proportions. By employing the techniques discussed in this guide, you can confidently solve real-world scenarios involving these concepts. So, delve into the intriguing world of variations and enhance your problem-solving prowess today!

